Easy Way to Multiply Whole Numbers and Fractions

Table of Contents

Multiplying Fractions

Fractions is a subject area that takes time and hard work to grasp. This in turn can be quite a worry for students and teachers.

It is important that students should be comfortable with the following:

• Multiplying numbers together. (If not, get them practising with Times Tables with Emile)
• What a fraction is and what it represents.

Students should know:

• What a numerator is.
• What a denominator is.

It is perfectly possible to multiply fractions without knowing these labels, but students may struggle to follow your directions if they are unsure.

Students may find it useful if they also understand basic algebra so that you can explain the steps using variables.

Multiplying Fractions: Method

This method is quite easy to understand for any level simply, to multiply any two fractions we can first multiply the numerators and then multiply the denominators and thirdly rearrange the set up.

So what does this look like?

So keeping in line with the multiplying method we simply…

1. Multiply the numerators 2 and 4 which equals 8
2. Multiply the denominators 3 and 5 which equals 15
3. And then we can rearrange the set up so it looks like this…

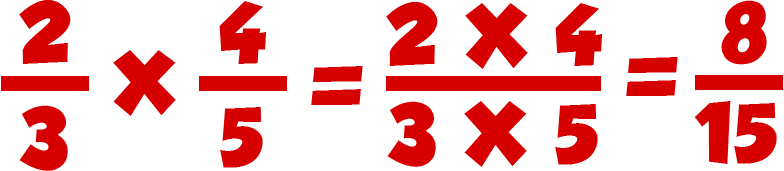
Keep in mind that this is a ROTE approach. So if you want to slowly and steadily introduce multiplying fractions to your class this is a good, solid starting point.

If your class start to pick this up quite quickly start thinking about working at a greater depth with this method by expressing two fractions together algebraically. Where the first fraction is a/b and the second fraction is c/d.

Visually Representing the Multiplication of Fractions

Here is a little tip to help visualise multiplying fractions using fraction squares.

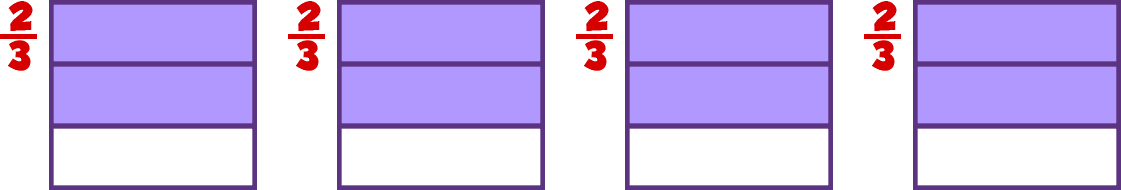
Let's take a look at an example: 2/3 x 1/2

As you can see the fraction square on the left has 2 purple sections shaded out of 3 equal sections. We, therefore, can determine that this purple area is two-thirds of the square.

Furthermore, we can see that the second fraction square represents a half of the shape. We can therefore, determine that the purple area shaded is half of the square.

Now if we overlay these coloured areas, we can see that they cover two sixths of the final fraction square. From this we can conclude that the answer is 2/6.

Note: This is an improper fraction and can be reduced down to 1/3.

Starting with Fractions & Whole Numbers

As your class grows more confident with multiplying fractions start introducing a new challenge of multiplying fractions by whole numbers.

With this example we are taking the equation 4x 2/3.

Which can be represented with a range of resources such as Lego and Number Blocks.

Think of different questions to ask your class like…

• How many thirds are there in total?

• How many purple sections?

• What does each purple section represent?

Using the Lego or Number blocks as a representation your pupils should be able to determine that there are 8 purple sections in the diagram.

Now explain to your class that each purple section is a third of a whole.

• Can your students figure out that there are eight thirds?

• Can they express this as a fraction?

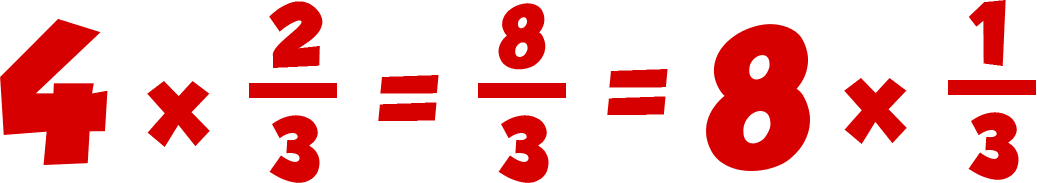
Extending from whole numbers to fractions

As your class progresses further make sure they know that whole numbers can be expressed as fractions.

• Ask your class how would this affect:

• We can then express the calculation as:

• Your students can then try to calculate this one on their own:

• Hopefully, this will lead your students to write the equations algebraically.

The Multiplying Fractions Rhyme

Equivalent Fractions

Equivalent Fractions as I am sure you are aware would have been covered in Years 3-5 of the National Curriculum.

Therefore with a Year 6 class, this will be a great opportunity to reinforce the concept.

•As an example take ²/₃ x ³/₄

•We can times the top numbers (numerators) together: 2 x 3 = 6

•We can times the bottom numbers (denominators) together: 3 x 4 = 12

Rearranging gives us:

Now 6/12 is equivalent to 1/2. So the most "satisfying" answer is:

Improper Fractions

An improper fraction is when the numerator (top number) is bigger than the denominator (bottom number)

For example, 5/4 is an improper fraction because 5 is bigger than 4.

 Tip: It is important for your students to know that when multiplying fractions, it is not uncommon to end up with improper fractions.

Here is an example of an answer being an improper fraction:

Explain to your class how the 8 is bigger than the 3 and now has made the fraction "top-heavy".

A better representation of this is to present the answer as a whole number and fraction together (simplified).

This is also, know as as Mixed Number.

Prime Factors

Prime factors can be used to good effect in multiplying fractions and what I really like is that it shows how learning about prime numbers can be useful. If I can show how something is useful then at least some of the students will be engaged! In the past, I have sold this approach as a cheat for doing difficult calculations.

Without a calculator, how do we determine the answer to:

Now the principle works on the same lines as this:

As long as we divide the top of the fraction and the bottom of the fraction by the same number, we won't affect the answer. (We know this from our work with equivalent fractions.)

Here we can remove (or cancel) the "3"s from the top and the bottom.

So now with this horrible calculation:

We can remove four of the "2"s and one of the "5"s from both top and bottom:

So looking back at the original calculation:

Emile and Multiplying Fractions

Emile

Active Learning is becoming a great way to resolve how to approach pupils engagement and understanding when it comes to learning. We here at Emile have utilised e-learning to work with Fractions for key stages 1 and 2.

• With Learn with Emile your pupils can develop their understanding on different maths topics and units with fun characters Emile, Aimee and Scrambler.
• In addition, your pupils can also, test their knowledge in class "vs. Multiplayer" or in "Single Player" mode in different worlds and story modes that can promote healthy competition and boost confidence in subject areas. All of course are created with the national curriculum in mind.
• Set informative tasks and homework with ease and TRACK each individual pupil's progress with an in-app database that is simple, quick and easy to use.

More Maths Blogs...

schroederript1991.blogspot.com

Source: https://www.emile-education.com/multiplying-fractions/

Share this post